When light propagates from air into glass or from glass in to air it may change its direction of travel. Snell's law reveals the relationship between the directions of travel in the two media.

n₁sinθ₁ = n₂sinθ₂

Consider light propagating in glass with index of refraction n₁ = 1.5 towards a glass-air boundary. If the angle the light makes with the normal to the boundary in the glass is θ₁, then the angle it makes in the air is given by

sinθ₂ = (n₁/n₂)sinθ₁ = 1.5 sinθ₁.

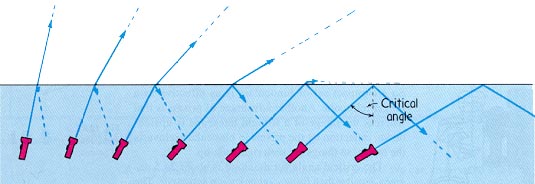
If sinθ₁ > (1/1.5) = 2/3, or θ₁ > 41.8^o, then sinθ₂ is greater than 1 and there is no solution for θ₂. The angle θ_c for which sinθ_c = n₂/n₁ is called the critical angle.  For angles greater than the critical angle there exists no solution for θ₂, and there is no refracted ray. The incident light is totally reflected, obeying the law of reflection. If n₂ = 1.5 and n₁ = 1 then the critical angle is θ_c = 41.8^o.

Total internal reflection occurs only if light travels from a medium of high index of refraction to a medium of low index of refraction. Let light travel from medium 1 into medium 2 and let n₁ > n₂. Then the critical angle θ_c is given by

sinθ_c = n₂/n₁

For angles greater than the critical angle the incident light is totally reflected, obeying the law of reflection.