Mechanical Waves

A wave pulse is a disturbance that moves through a medium. A periodic wave is a periodic disturbance that moves through a medium. The medium itself goes nowhere. The individual atoms and molecules in the medium oscillate about their equilibrium position, but their average position does not change. As they interact with their neighbors, they transfer some of their energy to them. The neighboring atoms in turn transfer this energy to their neighbors down the line. In this way the energy is transported throughout the medium, without the transport of any matter.
The animation on the right portrays a medium as a series of particles connected by springs. As one individual particle is disturbed, and then returns to its initial position, it transmits the disturbance to the next interconnected particle. This disturbance continues to be passed on to the next particle. The result is that energy is transported from one end of the medium to the other end of the medium without the actual transport of matter. Each particle returns to its original position.
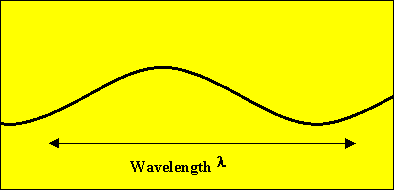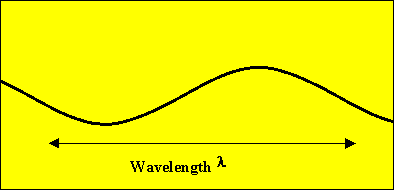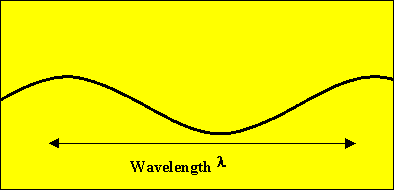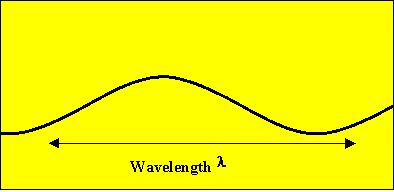
Periodic waves are characterized by a frequency, a wavelength, and by their speed. The wave frequency f is the oscillation frequency of the individual atoms or molecules. The period T = 1/f is the time it takes any particular atom or molecule to go through one complete cycle of its motion. The wavelength λ is the distance along the direction of propagation between two atoms which oscillate in phase. In a periodic wave, a pulse travels a distance of one wavelength λ in a time equal to one period T. The speed v of the wave can be expressed in terms of these quantities. v = λ/T = λf This relationship holds true for any periodic wave. If the individual atoms and molecules in the medium move with simple harmonic motion, the resulting periodic wave has a sinusoidal form. We call it a harmonic wave or a sinusoidal wave.

Transverse and longitudinal waves

If the displacement of the individual atoms or molecules is perpendicular to the direction the wave is traveling, the wave is called a transverse wave.

If the displacement is parallel to the direction of travel the wave is called a longitudinal wave or a compression wave.

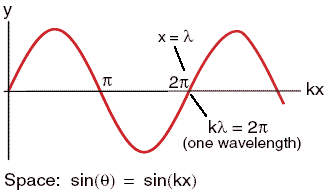
Consider a transverse harmonic wave traveling in the positive x-direction. Harmonic waves are sinusoidal waves. The displacement y of a particle in the medium is given as a function of x and t by

y(x,t) = Asin(kx - ωt + φ)

Here k is the wavenumber, k = 2π/λ, and ω = 2π/T = 2πf is the angular frequency of the wave. φ is called the phase constant.

	At a fixed time t the displacement y varies as a function of position x as y = Asin(kx) = Asin[(2π/λ)x] The phase constant φ is determined by the initial conditions of the motion. If at t = 0 and x = 0 the displacement y is zero, then φ = 0 or π. If at t = 0 and x = 0 the displacement has its maximum value, then φ = π/2. The quantity kx - ωt + φ is called the phase.
	At a fixed position x the displacement y varies as a function of time as y = Asin(ωt) = Asin[(2π/T)t] with a convenient choice of origin.

For the transverse harmonic wave y(x,t) = Asin(kx - ωt + φ) we may write

y(x,t) = Asin[(2π/λ)x - (2πf)t + φ] = Asin[(2π/λ)(x - λft) + φ]

or, using λf = v and 2π/λ = k,

y(x,t) = Asin[k(x - vt) + φ].

This wave travels into the positive x direction. Let φ = 0. Try to follow some point on the wave, for example a crest. For a crest we always have kx - vt = π/2. If the time t increases, the position x has to increase, to keep kx - vt = π/2.

For a transverse harmonic wave traveling in the negative x-direction we have

y(x,t) = Asin(kx + ωt + φ)= Asin(k(x + vt) + φ).

For a crest we always have kx + vt = π/2. If the time t increases, the position x has to decrease, to keep kx + vt = π/2.

To visualize the traveling wave, download this Excel spreadsheet. Macros must be enabled. The amplitude A of a wave is the magnitude of the maximum displacement of the individual particles from their equilibrium position. The energy carried by a wave is proportional to the square of its amplitude.

E is proportional to A²

The power P or energy per unit time delivered by the wave if it is absorbed is proportional to the square of its amplitude times its speed.

P is proportional to A²v

Interference

Two or more waves traveling in the same medium travel independently and can pass through each other. In regions where they overlap we only observe a single disturbance. We observe interference. When two or more waves interfere, the resulting displacement is equal to the vector sum of the individual displacements. If two waves with equal amplitudes overlap in phase, i.e. if crest meets crest and trough meets trough, then we observe a resultant wave with twice the amplitude. We have constructive interference. If the two overlapping waves, however, are completely out of phase, i.e. if crest meets trough, then the two waves cancel each other out completely. We have destructive interference.

Standing waves

Consider two waves with the same amplitude, frequency, and wavelength that are travelling in opposite directions on a string. Using the trigonometric identities sin(a + b) = sin(a)cos(b) + cos(a)sin(b) we write the resulting displacement of the string as a function of time as

y(x,t) = Asin(kx - ωt) + Asin(kx + ωt) = 2Asin(kx)cos(ωt).

This wave is no longer a traveling wave because the position and time dependence have been separated. All sections of the string oscillate either in phase or 180^o out of phase. The section of the string at position x oscillates with amplitude 2Asin(kx). No energy travels along the string. There are sections that oscillate with maximum amplitude and there are sections that do not oscillate at all. We have a standing wave.

Link: YouTube: Standing wave