Lagrangian problems, oscillations
Problem:
A light (assume massless) rod of length r is fixed at the origin, and a mass
M is attached to the other end, as shown.
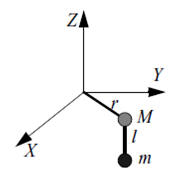
The rod is constrained to move in the XY-plane. A pendulum of length l
and mass m attached to M can oscillate in the YZ-plane. Use θ for the angle of
the rod in the XY-plane, and Φ for the angle of the
pendulum in the YZ-plane.
(a) Find Lagrange's equations for the system of the rod and pendulum in terms of
θ and Φ.
(b) Find the normal frequencies and normal modes of vibration for small
oscillations.
Solution:
- Concepts:
Lagrangian mechanics, L = T - U
- Reasoning:
We are asked to find the Lagrangian and solve the resulting equations of
motions for small oscillations.
- Details of the calculation:
TM = ½Mr2(dθ/dt)2, Tm = ½m((dx/dt)2
+ (dy/dt)2 + (dz/dt)2).
x = r cosθ, y = r sin θ + l sinΦ, z = -l cosΦ.
dx/dt = -r sinθ dθ/dt, dy/dt = r cosθ dθ/dt + l cosΦ dΦ/dt,
dz/dt = + l sinsΦ dΦ/dt,
Tm = ½m(r2(dθ/dt)2 + l2(dΦ/dt)2
+ 2rl cosθ (dθ/dt) cosΦ (dΦ/dt).
U = -mgl cosΦ
L = ½(M + m)r2(dθ/dt)2 + ½ml2(dΦ/dt)2
+ mrl cosθ cosΦ (dθ/dt)(dΦ/dt) + mgl cosΦ
∂L/∂(dθ/dt) = (M + m)r2(dθ/dt)
+ mrl cosθ cosΦ (dΦ/dt)
d/dt ∂L/∂(dθ/dt) = (M + m)r2d2θ/dt2 -
mrl[sinθ cosΦ (dΦ/dt)(dθ/dt) + cosθ sinΦ (dΦ/dt)2 - cosθ cosΦ d2Φ/dt2].
∂L/∂θ = -mrl sinθ cosΦ (dθ/dt)(dΦ/dt).
∂L/∂(dΦ/dt) = ml2(dΦ/dt) + mrl cosθ cosΦ (dθ/dt)
d/dt(∂L/∂(dΦ/dt)) = ml2d2Φ/dt2 -
mrl[sinθ cosΦ (dθ/dt)2 + cosθ sinΦ (dΦ/dt)(dθ/dt) - cosθ
cosΦ d2θ/dt2].
∂L/∂Φ = -mrl cosθ sinΦ (dθ/dt)(dΦ/dt) - mgl sinΦ.
Equations of motion:
(M + m)r2d2θ/dt2 - mrl[cosθ sinΦ (dΦ/dt)2
- cosθ cosΦ d2Φ/dt2] = 0.
d2Φ/dt2 - (r/l)[sinθ cosΦ (dθ/dt)2 - cosθ
cosΦ d2θ/dt2] + (g/l) sinΦ = 0.
(b) In the small angle approximation (θ << 1, Φ << 1)
we have the equations of motion
(M + m)r2d2θ/dt2
+ mrl d2Φ/dt2 = 0.
d2Φ/dt2 + (r/l)d2θ/dt2 + (g/l)Φ
= 0.
Assume Φ = Φ0 exp(iωt),
θ = θ0 exp(iωt) for a normal mode. Then
ω2(M + m)r2θ0 + ω2mrlΦ0
= 0, ω2(Φ0 + (r/l)θ0) - (g/l)Φ0
= 0.
There is only one oscillatory solution with ω not equal to zero.
θ0 = -(m/(m + M))(l/r)Φ0, ω2
= ((m + M)/M)(g/l).
Problem:
A simple pendulum of length l (massless string)
and mass m is suspended from a pivot point on the circumference of a
thin massless disc of radius a that rotates with a constant angular
velocity ω about its central axis as shown in the figure. Find the
equation of motion of the mass m in terms of the generalized variable θ.
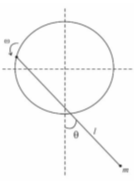
Solution:
- Concepts:
Lagrangian Mechanics
- Reasoning:
All forces except the forces of constraint are derivable from a potential.
The Lagrangian formalism is well suited for such a system.
- Details of the calculation:
The coordinates of the pivot point are X = a
cosωt, Y = a sinωt.
The coordinates of the mass m are x = X + l
sinθ, y = Y - l cosθ.
dx/dt = -aω sinωt + l cosθ dθ/dt, dy/dt = aω cosωt + l sinθ dθ/dt.
T = ½m((dx/dt)2 + (dy/dt)2)
= ½m[a2ω2 + l2(dθ/dt)2 + 2aωl(dθ/dt)(-sinωt cosθ
+ cosωt sinθ)]
= ½m[a2ω2 + l2(dθ/dt)2 + 2aωl(dθ/dt)
sin(θ - ωt)].
U = mgy = mg(a sinωt - l cosθ).
L = T - U.
Equation of motion:
d/dt ∂L/∂(dθ/dt) - ∂L/∂θ = 0.
∂L/∂(dθ/dt) = ml2(dθ/dt)+ maωl sin(θ - ωt).
d/dt(∂L/∂(dθ/dt)) = ml2d2θ/dt2 + maωl cos(θ
- ωt)(dθ/dt - ω).
∂L/∂θ = maωl (dθ/dt)cos(θ - ωt) - mgl sinθ.
ml2d2θ/dt2
+ maωl cos(θ - ωt)(dθ/dt - ω) - maωl (dθ/dt)cos(θ - ωt) + mgl sinθ = 0.
d2θ/dt2 - (a/l)ω2cos(θ - ωt) + (g/l)sinθ =
0
is the equation of motion.
Problem:
Consider the pendulum illustrated in the figure with l the
length of the string, m the mass of the ball, Fg the gravitational
force, and Φ the angular displacement. (You may assume the string
to be of fixed length and negligible mass). Use Lagrange's
equation to derive an equation of motion that neglects terms of order Φ3 and higher.

Solution:
- Concepts:
Lagrange's Equations
- Reasoning:
All forces except the forces of constraint are derivable from a potential.
The Lagrangian formalism is well suited for such a system. We are asked to
find the equation of motion.
- Details of the calculation:
T = ½ m l2(dΦ/dt)2,
U = -mglcos(Φ), L = T - U.
We have one generalized coordinate, Φ,
and one generalized velocity, dΦ/dt.
d/dt(∂L/∂(dq/dt)) -
∂L/∂q = 0.
The equation of motion therefore is ml2dΦ2/dt2 +
mglsin(Φ)
= 0,
or dΦ2/dt2 +
(g/l)sin(Φ) = 0.
sinΦ =
Φ - Φ3/3!
+ ...
Neglecting terms of order Φ3
and higher we have dΦ2/dt2
+ (g/l)Φ = 0.
Problem:
A simple pendulum of length with a bob of mass m is attached to a massless
support moving horizontally with constant acceleration a.
(a)
Compute the Lagrangian function.
(b) Write down Lagrange's equation of
motion.
(c) Simplify and compare the equation of motion to
that for a simple pendulum with a fixed (motionless) support.
Solution:
- Concepts:
Lagrange's equations
- Reasoning:
We are asked to obtain Lagrange's equation of motion.
- Details of the calculation:
(a) L = T - U.
T = ½m((dx/t)2
+(dy/dt)2).
x = ½at2 + sinθ, y = -b cosθ.
dx/dt
= at + cosθ(dθ/dt),
dy/dt = sinθ(dθ/dt).
T = ½m(b2(dθ/dt)2
+ a2t2 + 2at cosθ(dθ/dt)).
U = mgy = -mg cosθ.
L = ½m(b2
(dθ/dt)2
+ a2t2 + 2at cosθ (dθ/dt))
+ mg cosθ.
(b) ∂L/∂
(dθ/dt) =
mb2
(dθ/dt) +
mat bcosθ, d/dt(∂L/∂ (dθ/dt))
= mb2d2θ/dt2 -
mat bsinθ (dθ/dt) +
ma bcosθ,
∂L/∂θ = -matbsinθ (dθ/dt) -
mg bsinθ.
bd2θ/dt2 +
g sinθ + a cosθ = 0.
(c) sin(θ + θ0) = sinθ cosθ0
+ cosθ sinθ0.
Let g sinθ = c sinθ cosθ0, and a cosθ
= c cosθ sinθ0.
Then g = c cosθ0, and a = c sinθ0,
c = (g2 + a2)½, tanθ0 = a/g.
Define φ = θ + θ0. Then the equation of motion becomes
bdφ2/dt2
= -c sinφ. This equation becomes bdθ2/dt2 = -g sinθ
when a = 0.
For a simple pendulum we have bdθ2/dt2
= -g sinθ.
Problem:
A point particle of mass m slides without friction along a hoop of radius R
and mass M. The hoop rolls without slipping along a horizontal surface. What
is the frequency of small oscillations of the point mass, when it is close to
the bottom of the hoop?
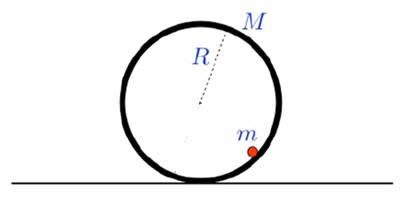
Solution:
- Concepts:
Lagrangian mechanics
- Reasoning:
All forces except the forces of constraint are derivable from a potential.
The Lagrangian formalism is well suited for such a system.
- Details of the calculation:
Let θ be the angle through which the hoop rotates and Φ be
the angle the line from the center of the hoop to the particle makes with
the vertical as shown below.
For the hoop X = Rθ. For the point mass x = Rθ + R sinΦ, y = -R cosΦ.
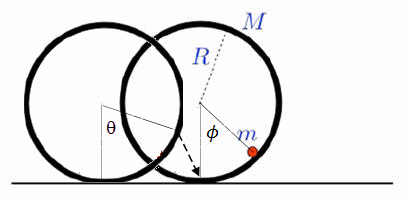
T = ½(MR2(dθ/dt)2 + I(dθ/dt)2) +
½m[R(dθ/dt) + R (dΦ/dt) cosΦ]2 + ½m[R (dΦ/dt) sinΦ]2
= (M + ½m)R2(dθ/dt)2 + ½mR2(dΦ/dt)2
+ mR2cosΦ(dθ/dt)(dΦ/dt).
U = - mgR cosΦ.
L = T - U.
∂L/∂(dθ/dt) = 2(M + ½m)R2(dθ/dt)+ mR2cosΦ(dΦ/dt) =
constant, because (dθ/dt) is cyclic.
∂L/∂θ = 0.
In the small angle approximation we then have
(2M + m)d2θ/dt2 + md2Φ/dt2 = 0.
d2θ/dt2 = -[m/(2M + m)]d2Φ/dt2.
In the small angle approximation we keep only terms to second order in the
small quantities in the Lagrangian or terms to first order in the small
quantities in the equations of motion.
L = (M + ½m)R2(dθ/dt)2 + ½mR2(dΦ/dt)2
+ mR2(dθ/dt)(dΦ/dt) - mgRΦ2/2.
∂L/∂(dΦ/dt) = mR2(dΦ/dt) + mR2(dθ/dt).
d/dt(∂L/∂(dΦ/dt)) = mR2d2Φ/dt2 + mR2d2θ/dt2.
∂L/∂Φ = -mgRΦ.
mR2d2Φ/dt2 + mR2d2θ/dt2
+ mgRΦ = 0.
d2Φ/dt2 + d2θ/dt2 + (g/R)Φ = 0.
d2Φ/dt2 -[m/(2M + m)]d2Φ/dt2 + (g/R)Φ
= 0.
[2M/(2M + m)]d2Φ/dt2 = -(g/R)Φ.
d2Φ/dt2 = -ω2Φ
ω2 = (g/R)(2M + m)/(2M).
Problem:
A thin rod of linear density ρ and length L is balanced on a cylindrical arc
of radius R as shown.
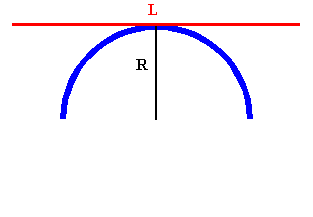
If one end of the rod is tapped, find the frequency of small oscillations for
the rod.
Solution:
- Concepts:
The Lagrangian formalism, small oscillations
- Reasoning:
We are asked to find the frequency of small oscillations.
- Details of the calculation:
Assume we have a very thin rod.
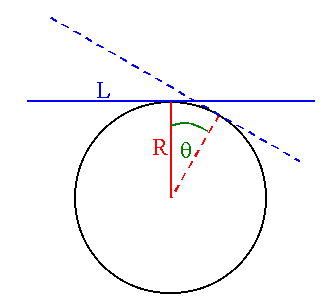
L = T - U.
- T = ½M((dX/dt)2 + (dY/dt)2) + ½I(dθ/dt)2.
U = MgY.
X and Y are the coordinates of the CM.
Let the origin of the coordinate system be the center of the circle in the
figure above.
X = R sinθ - Rθ cosθ.
Y = R cosθ + Rθ sinθ.
Small angle approximation: In L, keep only terms up to second order
in θ.
X = Rθ - Rθ = 0.
Y = R(1 - θ2/2) + Rθ2 = R(1 + θ2/2).
dX/dt = 0, dY/dt = Rθ dθ/dt.
Therefore
L = ½MR2θ2(dθ/dt)2 + ½M(L2/12)(dθ/dt)2
- MgR(1 + θ2/2)
≈ ½M(L2/12)(dθ/dt)2 - Mg R(1 + θ2/2).
Again: In the small angle approximation, where we only keep terms to second
order in the small quantities.
The equation of motion is
d2θ/dt2 = -(12gR/L2)θ,
which yields
ω = (12gR/L2)½,
independent of the mass of the rod.
Problem:
Consider an Atwood machine
with a massless pulley and two masses, m and M, which are attached at opposite
ends to a string of fixed length that is hung over the pulley. For this
Atwood machine the center of the pulley is supported by a spring of spring
constant k.
(a) Find the Lagrangian and the resulting equations of
motion.
(b) Find the equilibrium position of the pulley and its frequency of
oscillation. Consider your result in the limit m = M and discuss.
Solution:
- Concepts:
Small oscillation, Lagrangian mechanics.
- Reasoning
We are asked to find the Lagrangian and the equations of motion.
- Details of the calculation:
(a) Assume that the center of the pulley is a distance z measured in
the downward direction from the equilibrium length of the spring.
Assume that the distance from the center of the pulley for M is x measured
in the downward direction. For m the distance from the center of the
pulley downward is L - x, where L is a constant related to the length of the
string.
The kinetic energy of the two masses is
T = ½M(dz/dt + dx/dt)2 + ½m(dz/dt - dx/dt)2.
The potential energy of the two masses and the spring is
U = -Mg(z + x) - mg(z - x) + ½kZ2.
The Lagrangian is
L = ½M(dz/dt + dx/dt)2 + ½m(dz/dt - dx/dt)2 + Mg(z + x) + mg(z
- x) - ½kz2
= ½M((dz/dt)2+ (dx/dt)2 + 2(dz/dt)(dx/dt)) +
½m((dz/dt)2+ (dx/dt)2 - 2(dz/dt)(dx/dt))
+ Mg(z + x) + mg(z - x) - ½kz2.
The x-equation of motion is
(M - m)g
= (M + m)d2x/dt2 + (M - m)d2z/dt2.
The z-equation of motion is
(M + m)g - kz
= (M + m)d2z/dt2 + (M - m)d2x/dt2.
(b)
Solving for d2x/dt2 from the x-equation:
d2x/dt2
= (g - d2z/dt2)(M - m)/(M + m).
Substituting into the z-equation:
(M + m)g - kz
= (M + m)d2z/dt2 + (M - m)2(g - d2z/dt2)/(M + m).
[(M + m)2 - (M - m)2]d2z/dt2 =
(M + m)2g - (M + m)kz - (M - m)2g.
4Mm d2z/dt2 = 4Mmg - (M + m)kz.
d2z/dt2
= g - ((M + m)/(4Mm))kz.
The equilibrium position z0 of the center of the pulley requires
d2z/dt2 = 0. Therefore
z0 = (g/k)
4Mm/(M + m).
Let z' = z - z0. Then d2z'/dt2 = -
((M + m)/(4Mm))kz'.
z' = Cexp(iωt)
The frequency of oscillation is
ω2 = k(M +
m)/(4Mm) = k/(4μ). where μ = Mm/(M + m).
In the limit m = M the frequency of oscillation reduces to
ω2 = k/(2m), which is what you expect since neither
mass is accelerating due to gravity.







