Problem:

A hollow uncharged spherical conducting shell has an inner radius a and an outer radius b.  A positive point charge q is in the cavity at the center of the sphere.

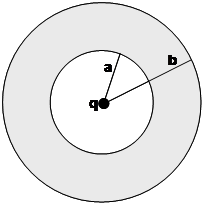
(a)  Find the charge on each surface of the conductor (surface a and surface b).
(b)  Find the electric field everywhere.
(c)  Find the potential everywhere, assuming that V = 0 at infinity.

Solution:

• Concepts:
  Gauss' law, properties of conductors
• Reasoning:
  The field due to a spherically symmetric charge distribution can be found from Gauss' law.
  The inside of a conductor is field free in electrostatics.
• Details of the calculation:
  (a)  Charge on surface a:  -q
  Charge on surface b:  q
  (b)   0 < r < a:  E = kq/r² (r/r)
  a < r < b:  E = 0
  r > b: E = kq/r² (r/r)
  (c)   r > b: V = kq/r
  a < r < b:  V = kq/b
  0 < r < a:  V = kq/b - kq/a + kq/r = kq(1/r + 1/b - 1/a)

Problem:

A spherical region of space of radius a contains a charge Q which is uniformly distributed within the volume
(a)  Use Gauss's law to determine the magnitude of the electric field at any radius r from the center of the sphere.
(b)  The total electrostatic energy of the sphere may be calculated from the electric field, using

U = (ε₀/2)∫_{all_space} E·E dV   (SI units).

Evaluate this expression for the uniformly charged sphere.
(c)  Calculate the work required to bring a test charge +q from infinity to the center of the sphere, using dW = F· dr = +qEdr.

Solution:

• Concepts:
  Gauss' law, electrostatic energy
• Reasoning:
  A radial field is produced by a spherically symmetric charge distribution.
• Details of the calculation:
  (a)  From Gauss' law we have E(r) = Q/(4πε₀r²) for r > a, and E(r) = ρr/(3ε₀) for r < a.  Here ρ = 3Q/4πa³.  Therefore E(r) =  Qr/(4πa³ε₀) for r < a.   At r = 0 we have E(r) = 0.
  (b)  U = (4πε₀/2)[ ∫₀^ar²dr Q²r²/(4πa³ε₀)² + ∫_a^∞r²dr Q²/(4πε₀r²)²]
  = (Q²/(8πε₀))[ ∫₀^ar⁴dr/a⁶ + ∫_a^∞dr /r²] = [Q²/(8πε₀)][1/(5a) + 1/a] = (3/5) Q²/(4πε₀a).
  (c)  W = q[∫₀^adr Qr/(4πa³ε₀) + ∫_a^∞dr Q/(4πε₀r²)] = [qQ/(4πε₀)][1/(2a) + 1/a]
  = 3qQ/(8πε₀ a).